A cylinder and a cone have equal bases. The height of the cylinder is 3 cm and the area of its base is 100 cm2 .The cone is placed upon the cylinder. Volume of the solid figure so formed is 500 cm3 . Find the total height of the figure.

Given height of cylinder , h = 3cm
Base area of cylinder , r² = 100cm² …………..(i)
Volume of solid = 500cm³
Given cylinder and cone have equal bases. the radii will be equal.
Radius of cone = radius of cylinder = r

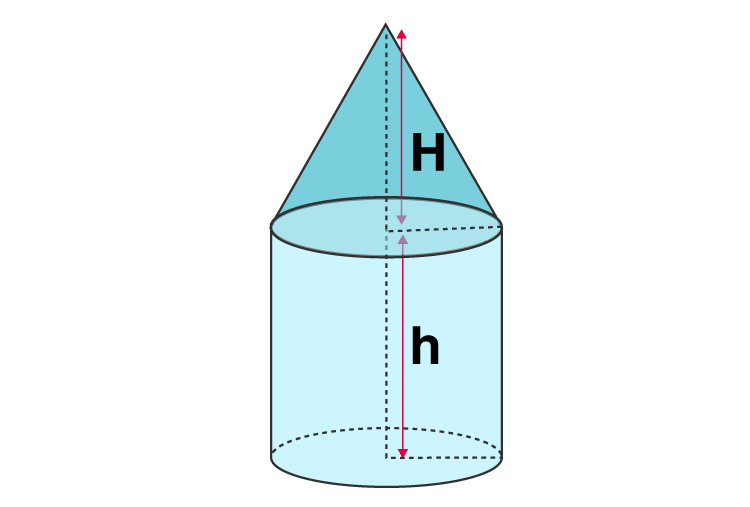
Let height of cone be H
Volume of solid = volume of cylinder + volume of cone
500 = r²h+(1/3) r²H
500 = 100×3+(1/3)×100×H [∵r² = 100, base area of cylinder and cone are equal]
500 = 300+(100/3)H
(100/3)H = 200
H = 3×200/100 = 6
Hence height of cone is 6cm.
Total height of figure = h+H
= 3+6 = 9cm
Hence the total height of the figure is 9cm.