Complete the following table to draw graph of the equations

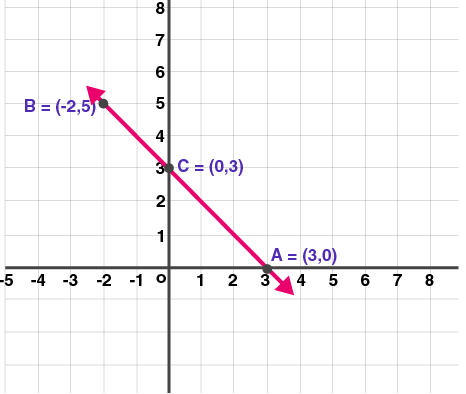
Solution:

(I) Given

x + y = 3 …. (i)
(i) Put value x=3 in equation (i)
We get, y = 3 – 3
⇒ y = 0
ii. Put value y = 5 in equation (i)
We get, x = 3 – 5
⇒ x = -2
iii. Put value y = 3 in equation (i)
We get, x = 3 – 3
⇒ x = 0
Now the table becomes,

x	3	-2	0
y	0	5	3
(x, y)	(3, 0)	(-2, 5)	(0, 3)

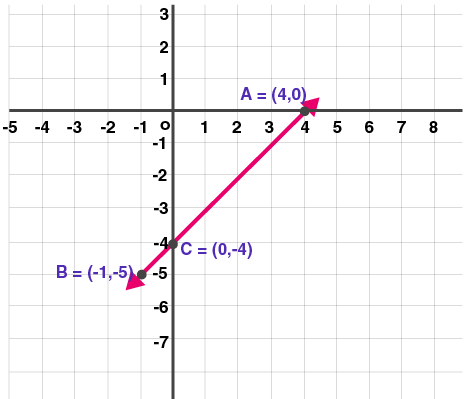
(2) Given

x – y = 4 ……. (ii)
i. Put value y = 0 in equation (ii)
we get, x = 4 – 0
⇒ x = 4
ii. Put value x = –1 in equation (ii)
we get, – y = 5
iii. Put value y = –4 in equation (ii)
we get, x = 4 + 4
⇒ x = 8

x	4	-1	0
y	0	-5	-4
(x, y)	(4, 0)	(-1, -5)	(0, -4)

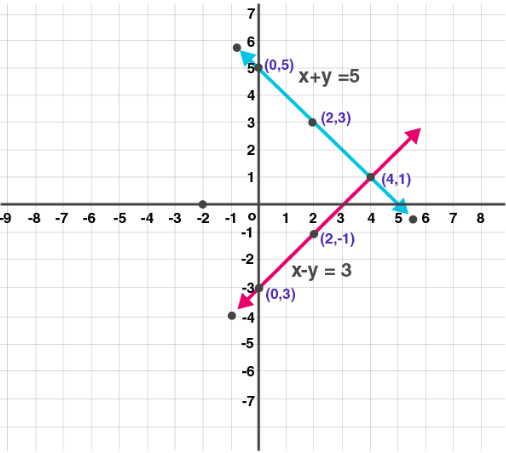
(2) x + y = 5; x – y = 3

Solution:

x + y = 5 …. (i)

x	0	2	4
y	5	3	1
(x, y)	(0, 5)	(2, 3)	(4, 1)

x – y = 3 …… (ii)

x	0	2	4
y	-3	-1	1
(x, y)	(0, -3)	(2, -1)	(4, 1)

Calculating intersecting point
x + y = 5
x – y = 3
which implies
2x = 8
x = 8/2
x = 4
Putting x= 4 in equation (i)
4 + y = 5
y = 5 – 4
y = 1
Intersection Point (4,1)

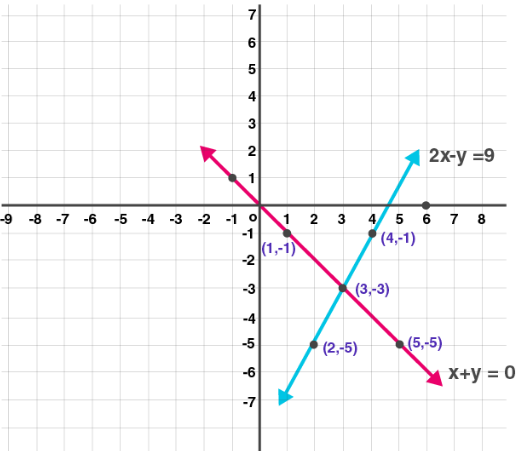
(3) x + y = 0; 2x – y = 9

Solution:

x + y = 0 …. (i)

x	1	3	5
y	-1	-3	-5
(x, y)	(1, -1)	(3, -3)	(5, -5)

2x – y = 9 …. (ii)

x	2	3	4
y	-5	-3	-1
(x, y)	(2, -5)	(3, -3)	(4, -1)

Calculating intersecting point
x + y = 0
2x – y = 9
3x = 9
x = 9/3
x = 3
Putting x= 3 in equation (i)
3 + y = 0
y = 0 – 3
y = -3
Intersection point (3, –3)

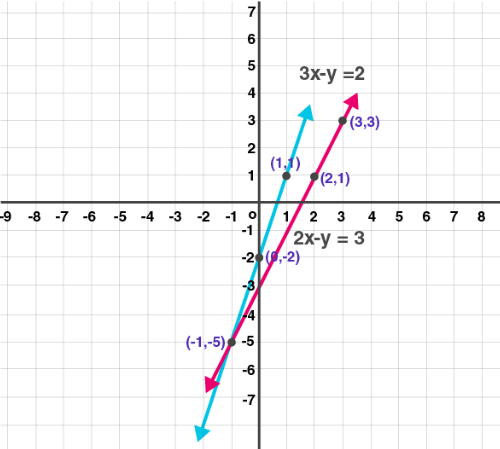
(4) 3x – y = 2; 2x – y = 3

Solution:

3x – y = 2 …… (i)

x	0	1	-1
Y	-2	1	-5
(x, y)	(0, -2)	(1, 1)	(-1, -5)

2x – y = 3 …… (ii)

x	3	2	-1
y	3	1	-5
(x, y)	(3, 3)	(2, 1)	(-1, -5)

Calculating intersecting point
3x – y = 2
– 2x + y = -3
x = -1
Putting x= –1 in equation (i)
3× –1 – y = 2
– 3 – y = 2
– y = 2 + 3
y = -5
Intersection point (–1, –5)

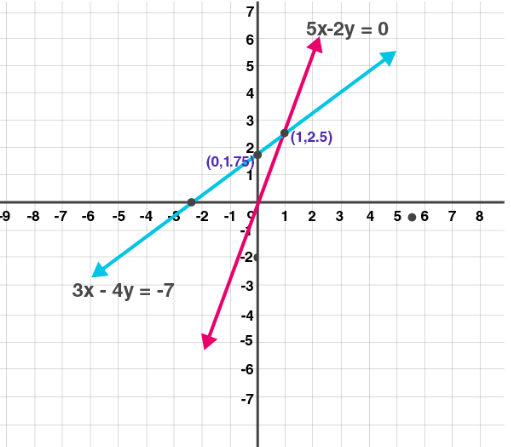
(5) 3x – 4y = –7; 5x – 2y = 0

Solution:

3x – 4y = 7 …. (i)
When x = 0, 4y = 7, y = 7/4
When y = 0, 3x = -7, x = -7/3
5x – 2y = 0 …… (ii)
When x = 0, y = 0
When x = 1, y = 5/2
Plotting both the graphs we get,
Calculating intersecting point
3x – 4y = -7
5x – 2y = 0
x = -1
Putting x= –1 in equation (i)
3 × –1 – y = 2
– 3 – y = 2
– y = 2 + 3
y = – 5
Intersection point (–1, –5)