In figure 3.83, M is the centre of the circle and seg KL is a tangent segment. If MK = 12, KL = 6√3 then find –

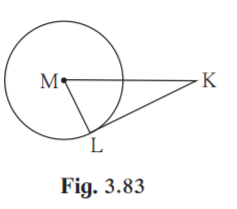
(1) Given KL is the tangent. ML is the radius.
MLK = 90˚ [Tangent theorem]
MK = 12, KL = 6√3
In MLK, ML²+KL² = MK² [Pythagoras theorem]
ML² = 12²-(6√3)² = 144-108 = 36
ML = 6
Hence radius of the circle is 6cm.
(2) ML = ½ MK
K = 30˚ [Converse of 30˚-60˚-90˚ theorem]
M = 180-(90+30) = 60˚ [Angle sum property]
Hence K = 30˚ and M = 60˚