is r and l(AB) = r, Prove that, ABOC is a square.
Construction: Draw OC and OB.
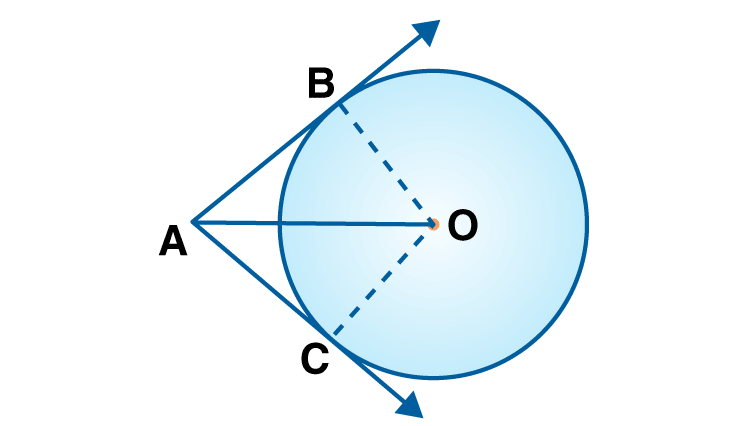
Proof:
Given AB and AC are the tangents and r is the radius of the circle.
AB = AC …….(i) [Two tangents from a common point are congruent]
OB = OC = r …(ii) [radii of same circle]
Given AB = r
AB = AC =OB = OC [From (i) and (ii)]
OBA = OCA = 90˚ [Tangent theorem]
ABOC is a square.
At AI Shiksha, we are driven by a singular mission – to democratize access to artificial intelligence education. We believe that AI is a transformative force that has the power to shape the future, and we are committed to making this cutting-edge technology accessible to everyone.