In figure 3.90, seg AB is a diameter of a circle with centre C. Line PQ is a tangent, which touches the circle at point T. seg AP line PQ and seg BQ line PQ. Prove that, seg CP seg CQ.

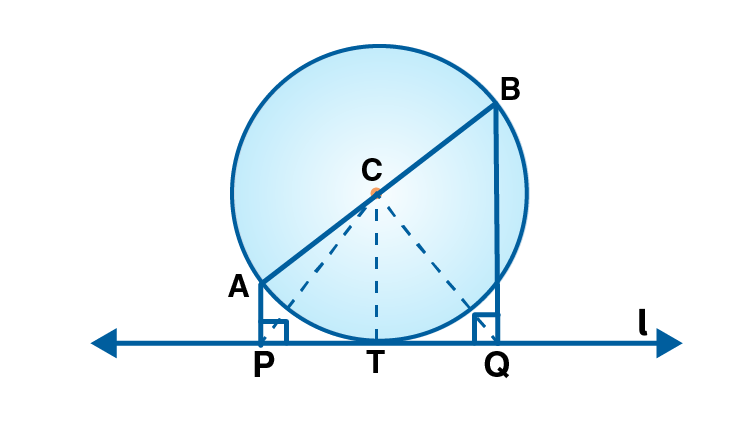
Given AB is the diameter of the circle with centre C.
PQ is the tangent.
AP PQ
BQPQ
To prove seg CP seg CQ
Construction: Join CP, CT and CQ.

Proof:
Since PQ is the tangent , CT PQ
Also AP PQ
BQ PQ
APCTBQ [Lines which are perpendicular to same lines are parallel]
AC/CB = PT/TQ …(i) [Property of three parallel lines and their transversals]
CB = AC [Radii of same circle]
(i) becomes PT/TQ = 1
PT = TQ ……..(ii)
In CTQ and CTP,
PT QT [From (ii)]
CT CT [Common side]
CTP CTQ [Given CT PQ]
CTP CTQ [SAS congruency test]
seg CP seg CQ [CPCT]
Hence proved.