In figure 7.43, A is the centre of the circle. ABC = 45° and AC = 7√2 cm. Find the area of segment BXC.

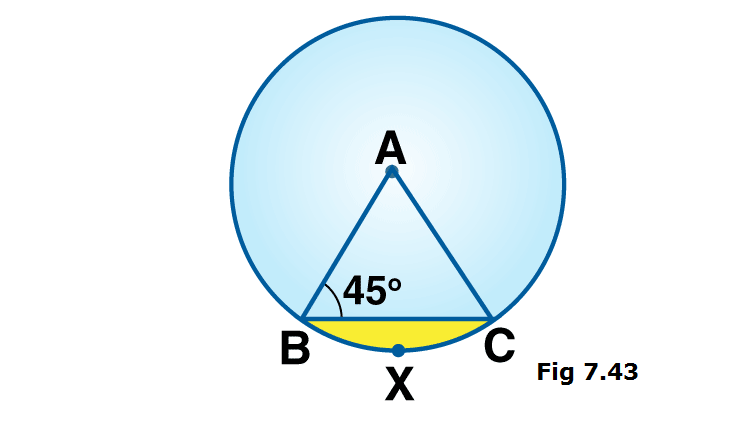
Given radius of circle, r = 7√2cm
AB = AC [radii of same circle]
ABC = ACB = 45° [isosceles triangle theorem]
InABC,
A = = 90˚ [Angle sum property of triangle]
Area of segment BXC = r²[(/360) – (sin/2)]
= (7√2)²[3.14×90/360 – (sin90˚)/2]
= 98×[(3.14/4)-(1/2)]
= 98×[0.785-0.5]
= 27.93cm²
Hence area of segment BXC is 27.93cm².