(1) What is d(O, P) = ? Why ?
(2) If d(O, Q) = 8 cm, where does the point Q lie ?
(3) If d(O,R) = 15 cm, How many locations of point R are line on line l ? At what distance will each of them be from point P ?
(1) Given radius = 9cm. Line l is the tangent to the circle.
d(O,P) = 9cm
OP is the radius of circle.
(2) d(O,Q) = 8cm.
d(O,Q) is less than radius. So Q will lie inside circle.
(3) Point R can be on two locations on line l.
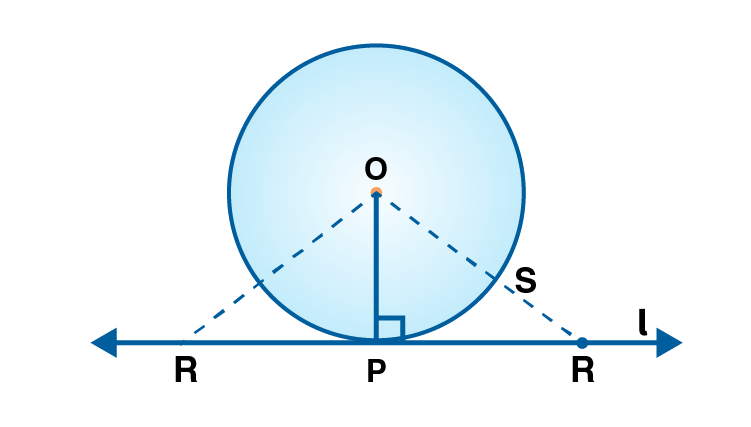
d(O,R ) = 15
InOPR, OPR = 90˚ [Tangent theorem]
OP2+PR2 = OR2 [Pythagoras theorem]
92+PR2 = 152
PR2 = 152-92
PR2 = 225-81 = 144
PR = √144 = 12
The point R will be at 12 cm distance from P.
At AI Shiksha, we are driven by a singular mission – to democratize access to artificial intelligence education. We believe that AI is a transformative force that has the power to shape the future, and we are committed to making this cutting-edge technology accessible to everyone.